Introduction
The subject of mathematics is considered to be essential to success in many post-secondary careers and in today’s labor market (Aughinbaugh, 2012; Kim et al., 2015). Further, the problem-solving skills that are gained through the study of mathematics can aid one’s full participation in our democratic system and the realization of one’s life aspirations (Allen, 2011; Moses & Cobb, 2002). Taking advanced mathematics courses in middle and secondary school improves mathematics achievement (Long et al., 2012; Stein et al., 2011), increases the likelihood of postsecondary school enrollment (Byun et al., 2015) and access to high paying science, technology, engineering, and mathematics (STEM) fields (Tyson et al., 2007; Warne et al., 2019).
A typical secondary school mathematics course sequence would start with Algebra I and continue with Geometry, Algebra II, Pre-Calculus, and Calculus. For academically prepared students, enrolling in Algebra I before secondary school— typically 7th or 8th grade—gives an advantage in increasing students’ likelihood of later enrolling in higher level mathematics courses such as Calculus and Advanced Placement (AP) mathematics courses during secondary school. However, when eligible students do not have access to Algebra I in middle school their likelihood of experiencing this enrichment is decreased. In the United States (US), only 60% of schools that serve 8th graders offer Algebra I and 24% of all 8th graders are enrolled in the course (USDOE, 2018). Black and Latino students are less likely to have access to Algebra I in middle school, especially when they attend segregated schools. Despite being 15% and 25% of the overall 8th-grade population, Black and Latino students make up only 10% and 18% of students enrolled in 8th grade Algebra I (Patrick et al., 2020).
Nationally, this disparity in access to Algebra I in middle school extends into the secondary school grades. Most secondary schools offered Algebra I (86%), Geometry (84%), and Algebra II (80%). However, advanced mathematics and Calculus were offered at fewer schools: 65% and 50%, respectively (CRDC, 2018). Further, these courses were offered at a lower rate (55%, 38%) among the approximately 5,000 secondary schools with high Black and Latino enrollment (defined in that report as schools with more than 75% Black and Latino student enrollment). This difference is greatest with respect to advanced mathematics and Calculus (CRDC, 2018). Schools with high Black and Latino enrollment are far less likely to offer advanced mathematics courses like Calculus compared to all secondary schools and even when they do, students often do not gain access to Algebra I until secondary school and are therefore not positioned to take advantage of advanced mathematics courses (CRDC, 2018).
Along these lines, English Learner (EL) students are far less likely to enroll in higher-level mathematics courses than their English proficient counterparts. In 2015–2016, ELs represented 6% of the secondary school population but only 2% of students enrolled in Calculus or beyond and 4% of students enrolled in Algebra II (CRDC, 2018). These students are often placed in lower mathematics tracks due to their language status (Gamoran, 2017), restricting access to higher-level content (Callahan, 2005). ELs are often pulled away from academic learning opportunities to receive English as a second language instruction as well (EdTrust, 2020).
Similarly, students with disabilities (SWDs) under the Individuals with Disabilities Education Act (IDEA) are less likely than their counterparts to enroll in higher-level mathematics courses. SWD accounted for about 12% of the student population in secondary school but they represented only 2% of Calculus enrollment in 2015–2016 (CRDC, 2018). Further illustrating the disparities apparent in STEM fields, secondary school SWDs acquire an approximately proportionate number of credits in English as other students but far fewer credits in mathematics or science than their counterparts (Schneiderwind & Johnson, 2020). Insufficient curricular and instructional adjustments to recognize and address the needs of SWDs often renders higher-level mathematics course content inaccessible (Moon et al., 2012).
This landscape study tracks mathematics course-taking trends in the state of New Jersey (NJ) for school years 2016–2017 through 2019–2020 by racial and economic segregation as well as by enrollment of ELs and SWDs. In addition, we highlight positive outliers which are highly segregated schools that also maintain high levels of enrollment in advanced mathematics courses.
New Jersey’s schools are highly segregated by race and income (Campbell & Payne, 2023). In 2018–2019, of 2,356 regular public schools, 407 enrolled 90% or more minority students, the majority of which were Black and Latino and 50% or more economically disadvantaged students based on free and reduced priced lunch status. Another 44 schools enrolled less than 50% economically disadvantaged students but 90% or more minority students. Nearly 300 schools (298) were racially mixed but enrolled 50% or more economically disadvantaged students (Campbell & Payne, 2023). As such, New Jersey has been considered one of the most segregated states in the country (Tractenberg et al., 2013).
The state is a local control state and, while learning standards and graduation requirements are coordinated at the state level, the sequence of courses, curriculum, pedagogy, and delivery of content are district- or school-level decisions. The New Jersey secondary school standards specify the mathematics that all students should study in order to be college and career ready. They are broken down into conceptual categories of Number & Quantity, Algebra, Functions, Modeling, Geometry, Statistics and Probability. Additional mathematics that students should learn in order to take advanced courses such as calculus, advanced statistics, or discrete mathematics are also identified such as applying trigonometry to general triangles (NJDOE, 2020).
Districts may handle the transition to secondary school in different ways in New Jersey. The K-7 standards contain the prerequisites to prepare students for Algebra I by 8th grade, and the standards are designed to permit districts’ existing policies concerning adoption of the course (NJDOE, 2020). The successful completion of Algebra I became a secondary school graduation requirement for all New Jersey students in 2012 with Geometry being added in 2014. There is also a requirement for a third year of mathematics that builds on the concepts and skills of the previous two courses, preparing students for postsecondary school and 21st century careers. These account for a minimum of 15 out of the 120 credits mandated for state graduation (see Table 1). Districts can choose what the third course entails.
Adapted from State of New Jersey (2018).
Algebra I is viewed as a gatekeeper to higher level courses and so many districts across the US have instituted reforms in order to enroll more traditionally underrepresented students in middle school. The impact of these reforms in various parts of the country has been mixed particularly for low-income students of color. A study of middle schoolers in North Carolina found that accelerated math placement of relatively low-skilled middle schoolers contributed to an increase in the number of students who enrolled in Pre-Calculus, particularly for female and non-low-income students (Domina et al., 2015; Dougherty et al., 2017). In Chicago, many low-achieving students were immersed in higher level academic coursework for the first time due to the Algebra-for-All policy. As a result, they experienced a rise in the ability levels of their classroom peers, but researchers found that simply requiring Algebra is insufficient to improve outcomes, even though students may benefit from having higher achieving peers in their classrooms (Nomi & Raudenbusch, 2014). Chicago subsequently implemented double-dose Algebra to address this issue, and research showed that offering extended instructional time and instructional supports to teachers was successful in improving learning for low achieving students (Nomi & Allensworth, 2009, 2010; Nomi et al., 2021). California also moved to make Algebra the accountability benchmark test for 8th grade mathematics. While this push increased enrollments for traditionally underserved students in California and nationally, research has found that it had negative average effects on students’ later math achievement in large districts and no benefits in small or medium districts (Domina et al., 2015). In 2014, San Francisco Unified School District introduced a new K-12 mathematics course sequence to address issues of inequity in mathematics learning. The curriculum delays Algebra I by one year and mandates all students take the same set of courses sequentially from 8th to 10th grade which has been subject to some controversy in the state (Fortin, 2021). Early research of this shift has found that participation in AP mathematics initially fell 15% (6 pp.) driven by declines in AP Calculus and among Asian/Pacific-Islander students. However, growing participation in acceleration options attenuated these reductions but large ethnic and racial gaps in advanced mathematics course-taking remained (Huffaker et al., 2023).
Advanced course enrollment can lead to increased post-secondary access but there are disparities. In one study, students who took an advanced academic mathematics curriculum in secondary school (Algebra II, Pre-Calculus, Trigonometry, Calculus) were found to be about 17 percentage points more likely to go to postsecondary school and 20 percentage points more likely to start postsecondary school at a 4-year institution by age 21 compared to those students whose highest mathematics class was Algebra I or Geometry (Byun et al., 2015). However, there are differences for students of color. A study using data from the High School Longitudinal Study of 2009 found sizable racial gaps in the likelihood of staying on (and getting on) the accelerated mathematics track in 9th grade. Interactions with parents and teachers positively predicted advanced mathematics course taking and, in some cases, interactions with teachers also reduced inequality in track persistence, whereas interactions with counselors increased such inequality (Irizarry, 2021). Students of color have more positive attitudes toward school when they have teachers of color (Keiser & Haider-Markel, 2022) and this representation can in turn increase student of color placement in higher-level coursework (Haider-Markel et al., 2022).
Further, a study of the relationship between Algebra I enrollment timing from 8th to 9th grade and sense of belonging in mathematics showed that enrolling and successfully passing 8th grade Algebra was protective for sense of belonging but this association differed by students’ race/ethnicity and the racial/ethnic incongruence between the mathematics class and school context (Morales-Chicas & Graham, 2021). In addition, a study using data from a large urban district investigated whether racial inequality in access to 8th-grade Algebra I is a reproduction of differences in prior opportunities to learn or whether patterns reflect an increase in inequality such that racial differences in access remain when controlling for academic background. The results point to patterns of reproduction of inequality in racially integrated schools, with some evidence of increasing inequality in predominantly Latino schools (Morton & Riegle-Crumb, 2019).
Mathematics course sequences can vary in different US states as well as in other countries. An analysis of mathematics course taking in the US found that there is a great deal of variation state by state. Among the nine states studied, a median of 27 percent of students went through the traditional course sequence, thirteen percent went through an accelerated track, taking Algebra I in 8th grade and 56 percent went through some other pathway (Schwartz, 2023). In the United Kingdom, students are not required to take mathematics after they pass their General Certificate of Secondary Education (GCSE) at 15 or 16 years old. However, Prime Minister Sunak called for changes to this practice so that students study mathematics until the end of secondary school in order to be better prepared for the modern job market. Many other countries including Australia, Canada, France, Germany, Finland, and Japan require students to take mathematics through age 18 but there is variability in how these countries perform on assessments (Schwartz, 2023).
Further, a study using Programme for International Student Assessment (PISA) data found that higher income students tend to enroll in more advanced mathematics coursework around the globe. This is a cross-country phenomenon and is not just restricted to the US. However, there are also major differences between countries such that in some systems, like France, Belgium, the Slovak Republic, Germany, Austria, and the Netherlands, there are larger between-school gaps and other systems, like Spain and Luxembourg, have greater within-school inequalities in content coverage (Schmidt et al., 2015). An analysis of the 2015 Trends in International Mathematics and Science Study (TIMSS) Advanced found that only 12.5 percent of all 12th graders in the US participated in the TIMSS Advanced for mathematics and the National Center for Education Statistics (NCES) found they scored below the international average in the subject (15 scale points lower). On average, boys outperformed girls and White students outperformed Black and Latino students on the test (Sparks, 2020). NCES also found wide variation in the topics covered by students’ mathematics courses. Students who took the highest-level AP Calculus BC course encountered more of the concepts on the exam. As such, they outperformed the international averages by 56 scale points in mathematics (Sparks, 2020).
One of the most important things we can do to ensure young people a healthy and successful young adulthood is to make sure they have a positive secondary school experience culminating in a strong diploma. A body of research on graduation pathways has developed over the past 10–15 years which can help to shape NJ’s educational policy discussions. One of the key findings from recent research is the critical importance of freshman year (or 9th grade). Students ending 9th grade year on track to move to the next grade are three times more likely to graduate and enroll in postsecondary school than off-track students. Freshman-on-track (FOT) status is a stronger predictor of a student’s odds of graduating than race, socioeconomic status, and prior achievement record combined (Allensworth, 2013).
The FOT lens has shaped the current analysis on mathematics course-taking, with implications for policies in the middle grades. It shapes the study in terms of likely trajectories. A key part of FOT research is performance in the core subject areas - failing no more than half of a core course. In NJ and most other states in the US, the core subject areas are those which have requirements for graduation and include mathematics, English Language Arts (ELA), science, social studies, and health/physical education. Our study centers on mathematics, one of the core subject areas, to broadly study trajectories in the state. We make the assumption that if large proportions of students in a school are enrolled in the standard or accelerated courses in each grade, then it is likely that the school tends to keep students on track in mathematics. However, moving past the on-track framework, the study further emphasizes acceleration as important for constructing student postsecondary success.
In addition to the focus on the extent to which students are on strong mathematics pathways our study is concerned with investigating the disparate distribution of this learning opportunity across the state. In their equity framework, Kim et al. (2021) define quantity as the distribution of programs available to students with respect to their number, length, and intensity and quality as the distribution of program type, efficacy, and rigor relative to the urbanicity and demographic composition of communities (see Table 2). The equity framework is applied here to study distribution of mathematics learning opportunities both in terms of intensity (enrollment rates) and rigor (accelerated trajectories likely to lead to advanced course-taking). As NJ is one of the most segregated states, understanding distribution of learning opportunities along the lines of segregation is imperative in the work to quantify and characterize what children lose or gain in this segregated context, which can help to inform policies within broader segregation remedies. The study identifies disparities through a total difference lens (Clemans-Cope et al., 2023) and analyzes mathematics course-taking, a learning opportunity, between categories of school segregation. Disparities research has argued for the importance of explicitly defining the disparity of interest and aligning measures with the selected disparity (Clemans-Cope et al., 2023). Thus, we feel it is important to explore advanced mathematics course taking in schools at varying levels of segregation as well as those that serve varying levels of ELs and SWDs.
We also examine racially and economically segregated high schools that appear to produce strong mathematics course-taking trends. That is, their trends differ from the overall observed patterns for these schools, and they are considered positive outliers. This type of analysis can be important for future research because effective practices and policies implemented in these schools can inform improvement efforts in schools serving similar populations. Studying positive outliers also demonstrate that well-implemented school practices and policies can indeed generate strong outcomes, regardless of the backgrounds of students served (Payne & Ortiz, 2017). They point us to what schools could do (Payne & Ortiz, 2017).
In this quantitative landscape study, we explore the following research questions:
-
What are mathematics course-taking trends by school composition (race, socioeconomic status, English Learners and students with disabilities) for NJ students in grades 8–12?
-
What kinds of disparities exist by school composition?
-
In terms of mathematics course-taking, what are the positive outliers in the state? What are their characteristics?
Methods
We used annual public education data released by the state’s Education Department. These data provide information on schools’ composition, course enrollment, school climate, and school resource indicators. Data from each year were merged into a single panel dataset and relevant measures extracted.
The segregation measures used in the study are measures of school composition. School composition measures are considered absolute measures of segregation because they quantify “how much students from one demographic group are exposed to (or isolated from) another demographic group within individual schools” (Monarrez et al., 2019, p. 3). Absolute measures of segregation contrast with relative measures of segregation, which take into account the underlying pool of students in the system from which schools draw enrollment and typically contain information about the levels of evenness or unevenness in the distribution of student groups across schools (Monarrez et al., 2019). Furthermore, because they are absolute, school composition measures of segregation do not indicate the mechanism or aspect of segregation being tested (Reardon & Owens, 2014). Although a more simplistic measure, school composition measures have been useful in landscape-type studies (Monarrez et al., 2019) which chart patterns of segregation (e.g., Frankenberg et al., 2019; Orfield, 2001; Orfield et al., 2016). Since our study comes in the context of active litigation around school segregation in NJ, the aims are to paint a picture of the landscape of how mathematics opportunities vary with segregation while making findings broadly accessible. It warrants the use of the more simplistic measure of segregation. In addition, the study was not designed to test hypothesized mechanisms through which segregation could affect mathematics educational opportunities.
To show disparities in high-leverage mathematics coursework, we categorized NJ secondary schools based on race/ethnicity of students enrolled and the socioeconomic status of their families (see Table 3). To operationalize the idea of “segregation,” we consider a school racially segregated if the percentage of White students is lower than 10%. In NJ, these are predominantly schools with majority Black and Latino students. On the other hand, if the percentage of White students is equal to or greater than 90%, the school is considered ‘White isolated’. Schools that do not belong to these two categories are considered ‘racially mixed.’ If the percentage of economically disadvantaged students is equal to or greater than 50%, the school is considered ‘economically segregated’. We selected levels for the categories based on common thresholds in influential segregation studies using composition measures either independently or as a component of unevenness or exposure measures (e.g., Orfield, 2001; Reardon & Owens, 2014).
We use the work of the National Center on Safe Supportive Learning Environments (NCSSLE) (2022) as a guide such that schools with a high percentage of ELs are defined as those with above 20%, schools with a medium percentage of ELs are defined as those with 5% to 20%, and schools with a low percentage of ELs are defined as those with below 5%. In New Jersey, as of 2019–2020, about 75% of schools were considered to have low concentrations of ELs, 15% medium concentrations of ELs, and 10% high concentrations of ELs. Similarly, we use the work of the Pew Research Center (2020) as a guide to identify schools with a high percentage of SWDs as those with more than 18%, schools with a medium percentage of SWDs as those with 14%–18%, and schools with a low percentage of SWDs are those less than 14%. In NJ, as of 2019–2020, more schools have low percentages of SWDs (41%) while a comparable number of schools enroll medium (31%) and high percentages of SWDs (29%).
NJDOE data releases include counts of students enrolled in middle and secondary school courses by grade level such as Algebra I, Geometry, Algebra II, Pre-Calculus, Calculus, Statistics, and Other Math. No direct measure of offering 8th grade Algebra I was available in the dataset. Schools were coded as offering Algebra I in 8th grade if they had non-zero enrollment in the 8th grade Algebra I course. Enrollment rates in each course for each grade were calculated based on the total number of students enrolled in the grade. Enrollment rates are reported by grade level beginning with 9th grade. The cross-sectional approach can be thought of in two ways. From one perspective, it shows the mathematics course-taking of a given grade over time. Looking at the cross-sections of all grades together approximates the likely mathematics trajectories of students throughout secondary school. The standard mathematics course-taking expectation in the US is that students take Algebra I, Geometry, Algebra II, after which they would take at least one advanced mathematics course such as Pre-Calculus or Calculus. It becomes easier for students to take multiple advanced mathematics courses if they started the secondary sequence earlier in the middle grades.
The first goal of the analysis was to examine the patterns in course enrollment rates by school segregation and EL and SWD composition. To account for trends over time, the analysis shows the four-year average enrollment rate by course and grade level for grades 9 through 12. Studying the grade cross-sections illuminates the typical trajectory of course-taking in each type of school.
Disparity ratios were calculated to quantify the difference in distribution of opportunities for acceleration in mathematics pathways. For example, if some secondary schools have higher rates of 9th graders taking Geometry, then they would be in contexts where they attract students who attended middle schools which offered Algebra I in 8th grade. Enrollment rates by course and grade level were used to calculate disparity ratios (Equation 1). For segregation disparity ratios, since in most cases White Isolated schools had the highest rates of course-taking in the mathematics courses along the accelerated trajectory, ratios were calculated with the rates for White Isolated schools as the base. Enrollment rates for low EL and Low SWD schools were the base for those disparity ratios as those categories typically had higher enrollment rates. Disparity ratios were calculated for the intersection of school segregation with EL and SWD composition. For those ratios, the base is White Isolated and low EL or low SWD. Disparity ratios were calculated for 9th grade Geometry, 10th grade Algebra II, 11th grade Pre-Calculus or Calculus, and 12th grade Calculus, markers of accelerated mathematics course-taking.
There are limitations to using aggregate school-level data. The extent to which students are repeating courses is unknown, which could change how the trends can be interpreted. Further, counts of course-takers are not mutually exclusive given that students are counted in each subject for which they had an enrollment. Therefore, if many students are enrolled in multiple mathematics courses each year, we cannot tease that out in the analysis. Finally, this data cannot give insight into what comprises ‘Other Math’; it is a catch-all category and does not indicate the levels of courses included.
Findings
In this section, we discuss mathematics course-taking trends in middle (grades 6–8) and secondary school (grades 9–12). We also explore mathematics course-taking trends by EL and SWD composition. Lastly, we identify positive outliers in terms of segregation - schools that are segregated and enroll students in advanced mathematics courses at rates much higher than the average for their category.
In grades 6–8, students in racially and economically segregated schools in NJ have far lower rates of access to Algebra I (measured by zero enrollment) than those in other schools (Fig. 1). As of 2019, 70% of racially and economically mixed schools with 6th–8th grades and 80% of White isolated schools offered the course. However, among schools segregated racially and economically, only 48% offered Algebra I in 6th–8th grade. This is consistent with the research literature on the national level finding a similar disparity (Peters & Carter, 2021). While we cannot examine specific middle to secondary school transition patterns, such as whether students from segregated middle schools will subsequently enroll in segregated secondary schools, we assume that since school segregation is largely (though not entirely) driven by neighborhood segregation, many students will enroll in secondary schools that are similarly segregated. In the remaining sections we present mathematics course enrollment patterns in secondary schools which show persistent disparities in advanced course enrollment. We hypothesize that at least some of the disparities are related to gaps in access to Algebra I earlier in 6th–8th grade.
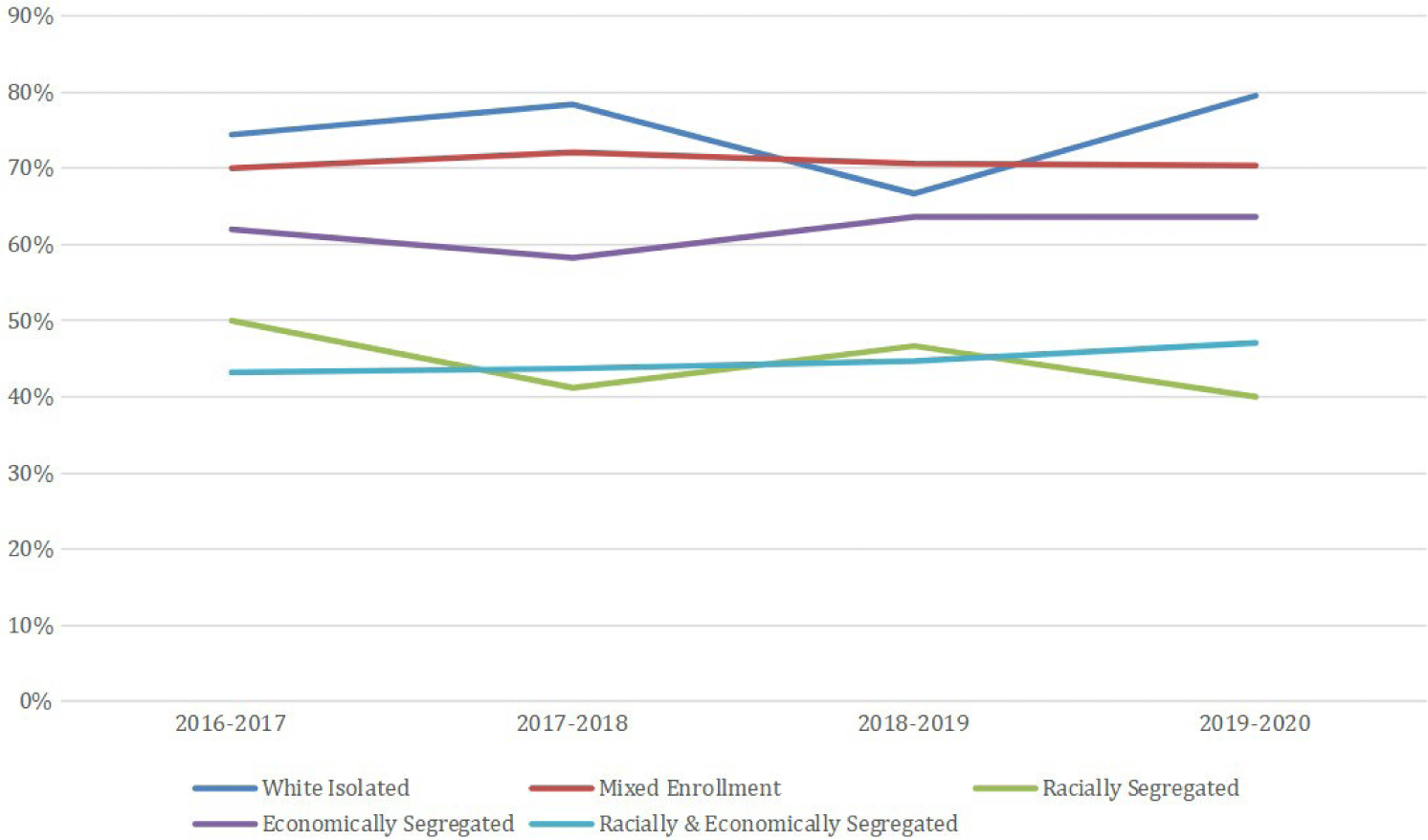
With mathematics course enrollment rates exceeding 100% each year, we can infer that some students are taking multiple mathematics courses in 9th grade across the school types. The highest rates of ‘Other Math’ enrollment during 9th grade are found in schools segregated racially and economically. As described, one limitation is that we cannot tell the extent to which this reflects innovative course-taking versus remedial course-taking. As of 2019–2020, ‘Other Math’ enrollment was 16% in schools segregated racially and economically, 18% in schools segregated economically, and 12% in mixed enrollment schools.
Nonetheless, on average, over four years, the differences in 9th grade Algebra I enrollment rates tell a story of advantage for students in White isolated and mixed enrollment schools (Table 4). Taking mixed enrollment schools as a comparison, proportionally more students are further along in the mathematics sequence. Freshmen in mixed enrollment schools are taking Geometry, the next course in the mathematics sequence, at higher rates than freshmen in the segregated school categories (11 percentage point difference). In schools segregated racially and/or economically, less than 20% of 9th graders are enrolled in Geometry (17%–19%) compared to 29% at mixed enrollment and White isolated schools.
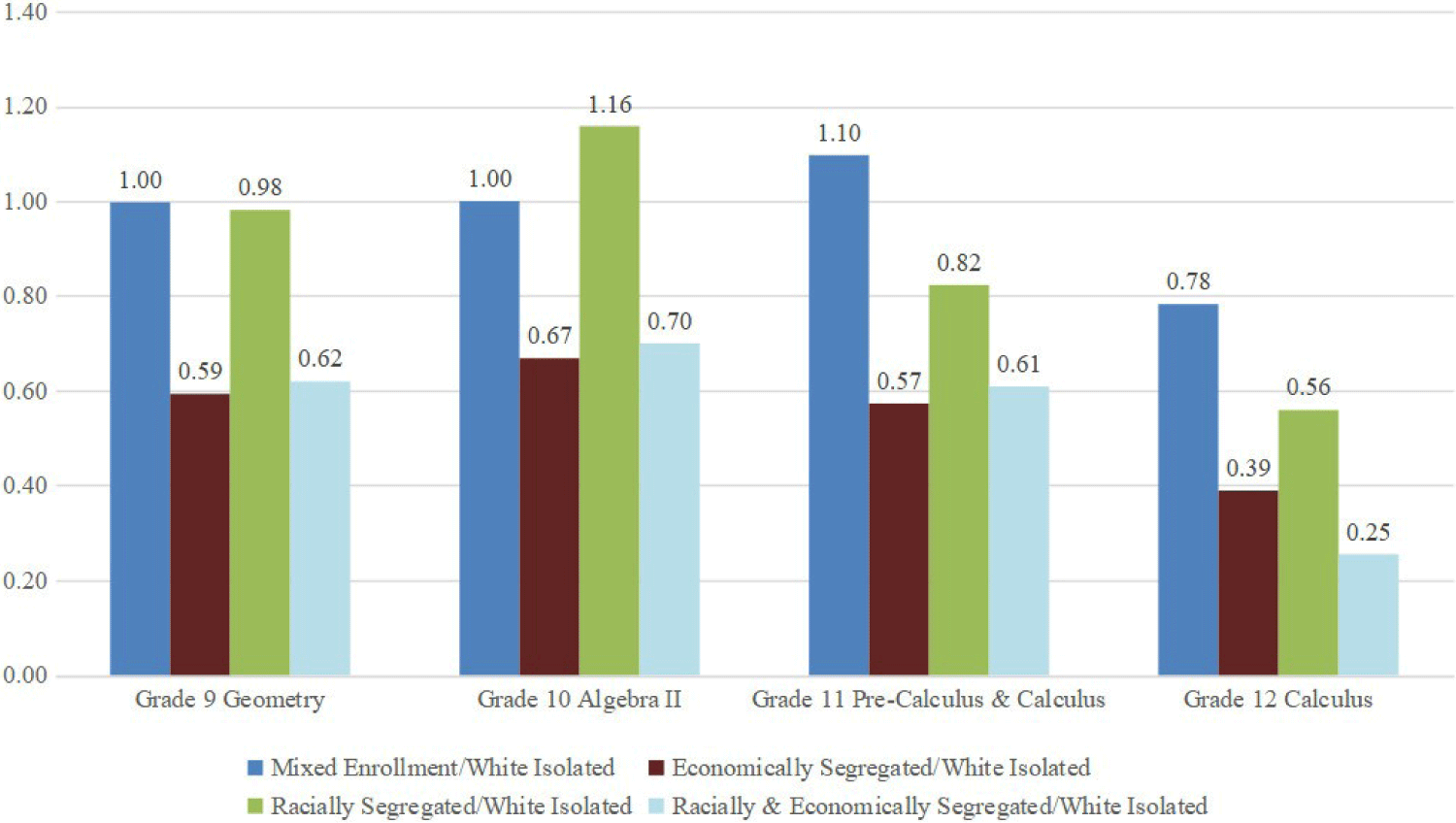
When we examine disparity ratios, by 2019–2020, students in mixed enrollment schools are just as likely to enroll in geometry in 9th grade as those in White isolated schools (Fig. 2). Interestingly, the same is true for those in racially segregated schools. The 10 racially segregated schools have a mix of schools which are predominantly Asian (2), predominantly Black and Latino (7), or predominantly Latino and Asian (1). One predominantly Asian school has all 9th graders enrolled in Geometry. The second predominantly Asian school has half of 9th graders enrolled in Geometry. One school with majority Black and Latino enrollment in Camden City School District, has about a third of its 9th graders taking Geometry. These three schools appear to drive up the 9th grade Geometry enrollment rate for the racially segregated category in 2019–2020. Since only 10 schools are in this category, averages can be volatile. Students in racially and economically segregated schools or economically segregated schools are about half as likely to enroll in geometry in 9th grade as those in White isolated schools suggesting that socioeconomic background is an important factor in the distribution of advanced mathematics coursework.
Looking at the four-year average, while 69% of students in schools segregated racially and economically and 70% in schools segregated economically are taking Algebra I in 9th grade, only about 59% of students in mixed enrollment schools are doing so. Students in racially and/or economically segregated schools tend to be on a standard pathway and not accelerated. Although the disparity in 9th grade Algebra I and Geometry course enrollments provides important insight, we do not know what courses are included in ‘Other Math’ and how this may improve or worsen the conclusions.
In 10th grade, the roughly 10-point gap remains with respect to taking the accelerated mathematics pathway between segregated schools and mixed enrollment or White isolated schools (Table 4). In racially and economically mixed schools, just over half of students take Geometry (54%) while nearly one-third (31%) of students have moved on to take Algebra II. In racially and economically segregated schools (66%) and economically segregated schools (62%), proportionally more 10th graders are enrolled in Geometry and only about 20% are enrolled in Algebra II. Taking Algebra II in 10th grade would signal some acceleration in the mathematics sequence and here, the gap remains at 10 percentage points. In schools segregated economically, there seems to be a reliance on the ‘Other Math’ category reflected by the 21% of students enrolled on average over four years.
Similar to Geometry in 9th grade, disparity ratios suggest that students in mixed enrollment schools are just as likely as those in White isolated schools to enroll in Algebra II in 10th grade (Fig. 2). Further, those in racially segregated schools are more likely to enroll in the course than those in White isolated schools. However, those in economically and racially and economically segregated schools are less than half as likely to enroll in the course as those in White isolated schools.
A clear gap in taking accelerated mathematics is solidified by 11th grade when, on average across four years, around 27% of students in mixed enrollment schools are taking Pre-Calculus while 16% in schools segregated racially and economically and schools segregated economically are doing so (Table 4). Less than half (44%) of students in 11th grade take Algebra II in non-segregated schools. Thus, the roughly 10-point gap in taking more advanced mathematics courses along the trajectory remains. Considering Pre-Calculus and Calculus together, the gap is 15 points. In mixed enrollment schools, 34% of juniors are taking either Pre-Calculus or Calculus compared to 19% in schools segregated racially and economically and schools segregated economically.
Instead, students in schools segregated racially and economically appear to take the standard mathematics sequence described earlier with little acceleration. A little more than half (62%) enrolled in Algebra II in 11th grade. Some also seem to be a step behind the standard sequence with 18% of juniors taking either Algebra I or Geometry. The same is true in schools segregated economically – 49% of juniors are taking Algebra II and 20% are still taking Algebra I or Geometry.
When examining disparity ratios, students in mixed enrollment schools are more likely to enroll in Pre-Calculus and Calculus in 11th grade than those in White isolated schools (Fig. 2). Further, those in racially segregated schools are almost as likely to enroll in the courses. However, those in economically and racially and economically segregated schools are a little less than half as likely to enroll in the course.
Research has demonstrated that taking a fourth year of mathematics is associated with success in postsecondary school and with higher earnings (Poulsen, 2019), though a causal relationship is not established. Overall, on average across four years, mathematics enrollment is high during 12th grade in NJ, with rates ranging from 78% to 100% across all school types shown, but a 17-point gap in taking advanced courses persists between segregated school categories and mixed enrollment or White isolated schools (Table 4). Rates of mathematics course-taking in more advanced courses such as Pre-Calculus and Calculus in schools segregated racially and economically or segregated economically alone do not appear to catch up to those in White isolated schools. Here, racially segregated schools are also behind. On average across four years, in schools segregated racially and economically, 37% of seniors take Pre-Calculus, Calculus, or Statistics; 38% do so in schools segregated racially and in schools segregated economically; and 59% in mixed enrollment schools; and 71% in White isolated schools, reflecting a gap as wide as 34 percentage points. Looking only at the measure of Pre-Calculus or Calculus enrollment, 30% of seniors in schools segregated racially and economically took either course; 27% in schools segregated economically; 23% in schools segregated racially while 40% and 48% did so in mixed enrollment and White isolated schools respectively.
Therefore, with persistent mathematics course-taking disparities from 8th grade through 12th grade, we have reason to believe that an advantage is solidified for students in non-segregated schools. Still, the proportion of students in segregated schools taking advanced mathematics opportunities is not insubstantial (e.g., 29% taking Pre-Calculus or Calculus in 12th grade) and this should encourage a drive towards closing the gaps.
According to disparity ratios, in mixed enrollment schools, students are not quite on par with those in White isolated schools with their enrollment in 12th grade Calculus (Fig. 2). Those in racially segregated schools are about half as likely and those in economically and racially and economically segregated schools are far less likely to enroll in the course. This suggests that while mixed enrollment and racially segregated schools start secondary school with similar enrollment trends as White isolated schools, they do not continue on the same trajectory throughout secondary school.
Now, we consider mathematics course-taking trends in schools by composition of ELs. The national average of public-school students in the US classified as ELs in 2018 was 10.2%, or 5 million students (NCES, 2021). In New Jersey, it was 7% or 93,000 students (NJDOE, 2021).
When comparing grade 9 mathematics course-taking trends across the three EL composition school types, on average across four years, all tend to enroll students in Algebra I, but there are subtle differences (Table 5). In high and low EL schools, proportionally fewer students enroll in Algebra I (60%) than medium EL schools (71%). Less than a quarter of students are enrolled in Geometry in high and medium EL schools suggesting modest rates of accelerating students in contrast to low EL schools in which nearly 30% of freshmen are enrolled in Geometry indicating greater tendency for students to accelerate in these schools. The trends for low EL schools mirror the trends for racially mixed and White isolated schools. Interestingly, nearly 20% of students are enrolled in ‘Other Math’ in high and medium EL schools. This will be important to study further because, as the literature shows, there is a tendency for EL students to be enrolled in remedial mathematics courses in secondary school (Gamoran, 2017).
In 10th grade, a similar lag between high EL and low EL schools shows up when investigating Algebra II enrollment (Table 5). On average across four years, the enrollment rate is 21% in high EL schools compared to 31% in low EL schools. This is true, even with comparable Geometry enrollment rates - 57% in high EL schools and 56% in low EL schools. Medium EL schools are also behind low EL schools, showing evidence of a more standard mathematics trajectory - 63% of 10th graders take Geometry and 24% take Algebra II. The differences in enrollment rates between medium and low EL schools are smaller, though present. The general takeaway is that higher EL composition seems associated with a standard mathematics course-taking sequence while low EL schools have greater acceleration.
Grade 11 patterns show further widening of the gaps. High EL schools enroll 19% of students in Pre-Calculus or Calculus and medium EL schools enroll 22% in the courses compared to 32% in low EL schools. This is partly because about 7% of students in low EL schools are taking Calculus in 11th grade on average. Just examining Pre-Calculus alone, the enrollment rates are 16%, 19%, and 25% in high, medium, and low EL schools respectively. As the EL population increases in schools, the chances of experiencing an accelerated mathematics sequence diminishes.
Low EL schools have a clearer tendency to enroll seniors in more advanced mathematics course-taking with 57% of seniors taking either Pre-Calculus, Calculus, or Statistics compared to 43% in medium EL and 39% in high EL doing the same. Between low and high EL, the gap is 19 percentage points. On average, proportionally far more students in low EL schools are taking Calculus by senior year – 24% compared to 14% in high EL and 12% in medium EL – and Statistics – 18% in low EL schools compared to 12% in medium EL and 9% in high EL schools. Still, despite the 9–11th grade mathematics course-taking patterns in high EL schools, these schools manage to enroll proportionally more students in Calculus in 12th grade than medium EL schools – a 2 percentage point difference.
In general, when considering the EL composition in schools, low EL schools mirror the patterns in White isolated schools in that they both appear to accelerate about one-third or more of their students each grade. In contrast, less than 20% to 25% of students in low and medium EL schools seem to benefit from mathematics acceleration.
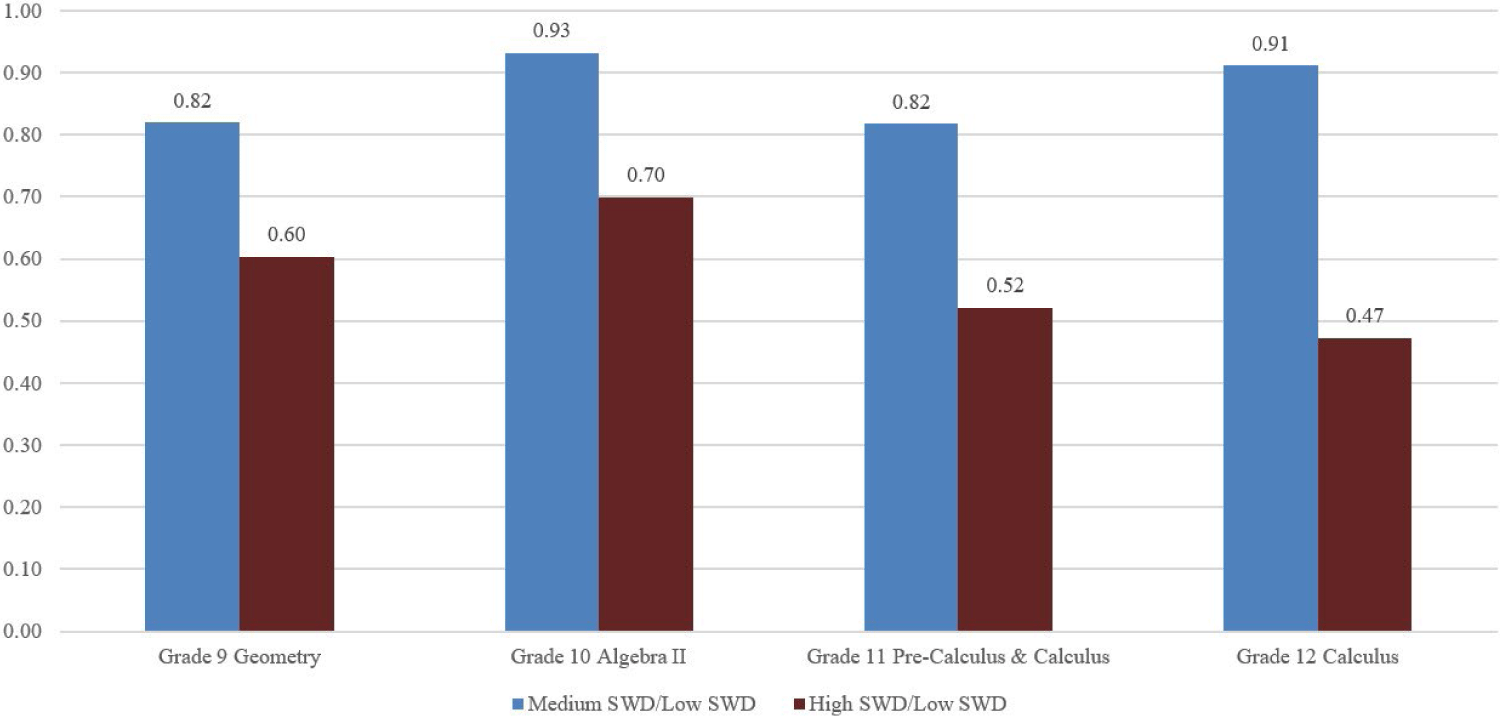
The 2019–2020 disparity ratios, however, give evidence of a shift for high EL schools (Fig. 3). The disparity ratios confirm that students in medium and high EL schools are a little less likely to experience accelerated mathematics pathways compared to low EL schools. However, relative to low EL schools, on most measures high EL schools have a smaller disparity than medium EL schools. In 12th grade, students in medium EL schools are just about half (0.54) as likely to enroll in Calculus than low EL schools whereas the disparity for high EL schools is 0.70.
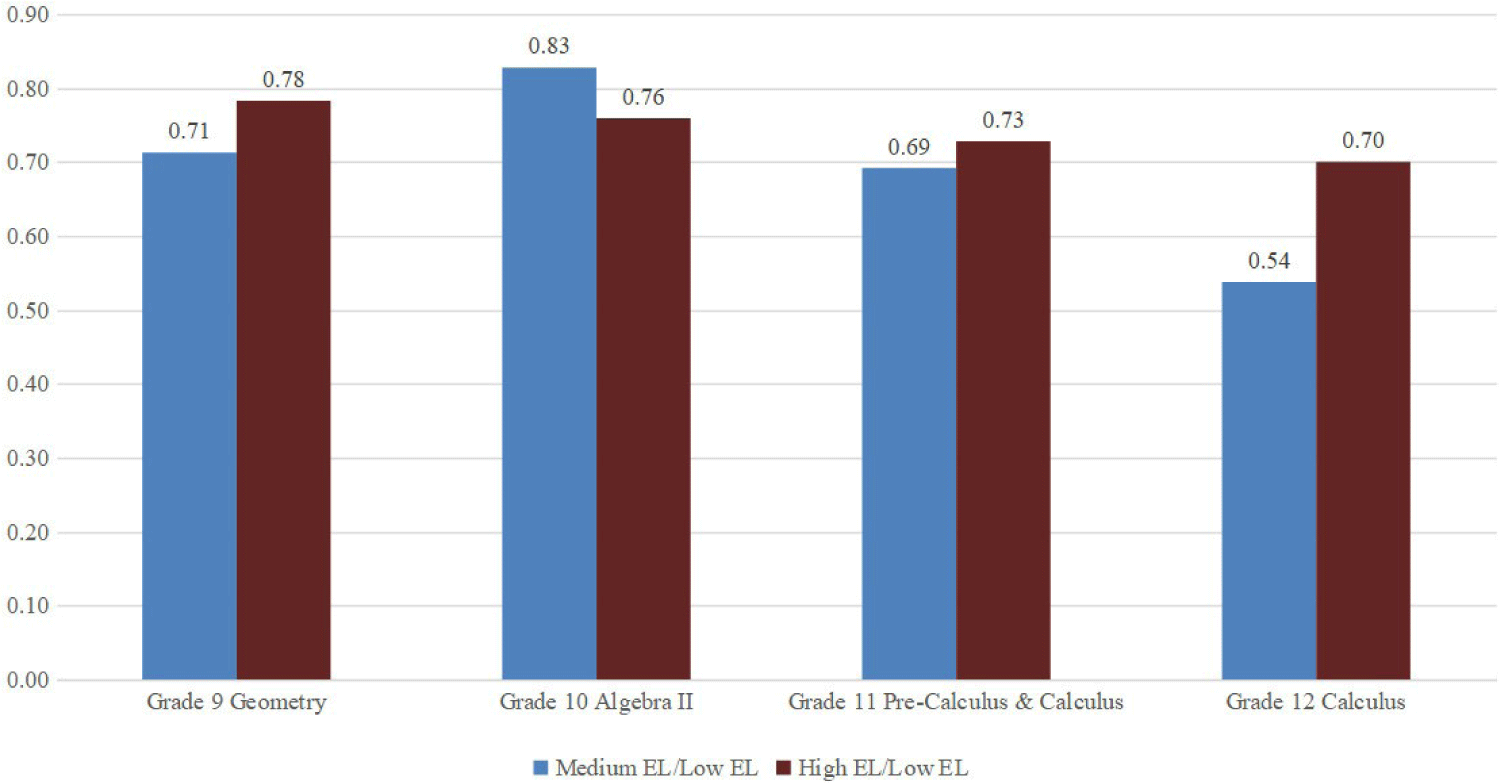
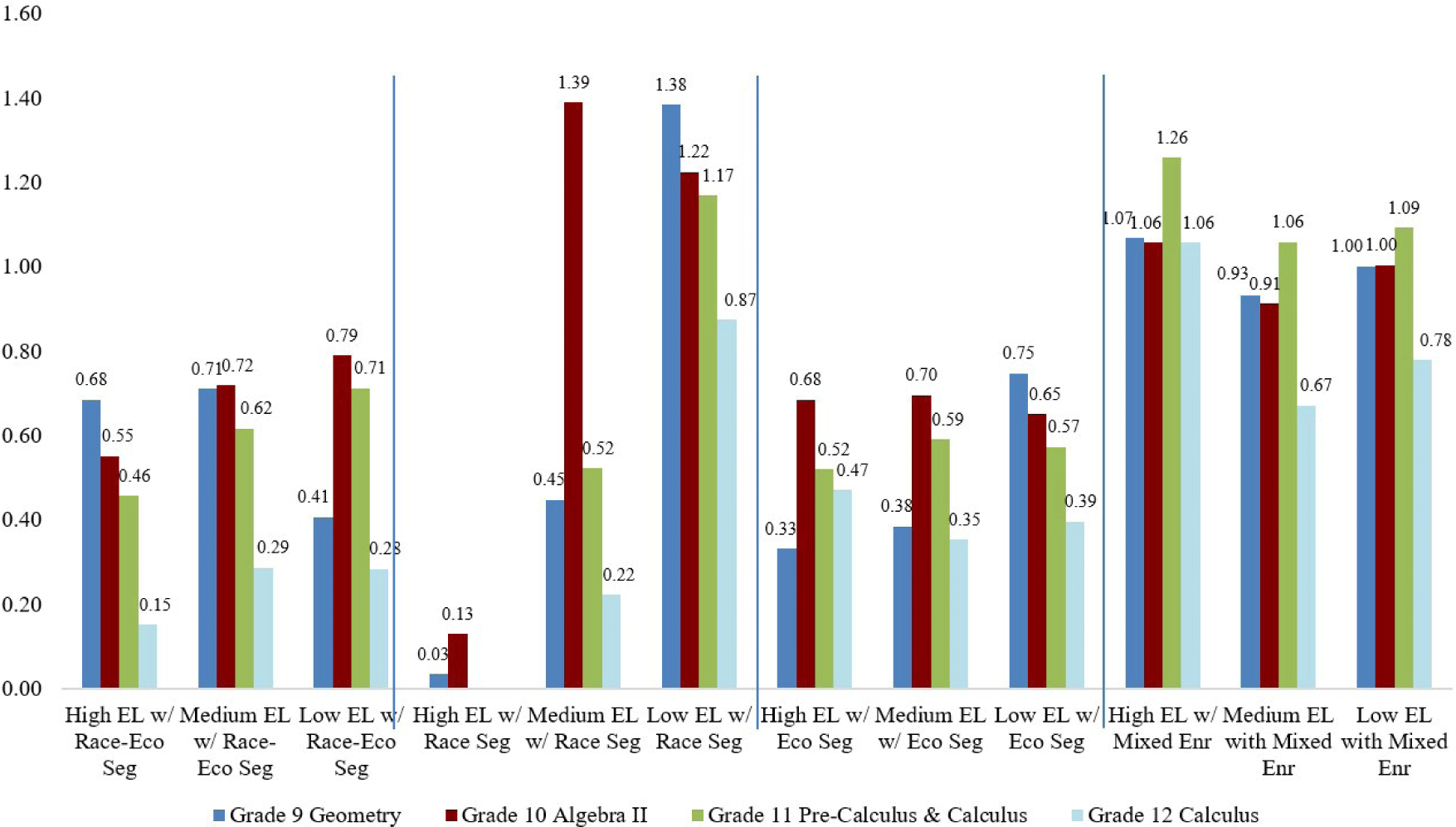
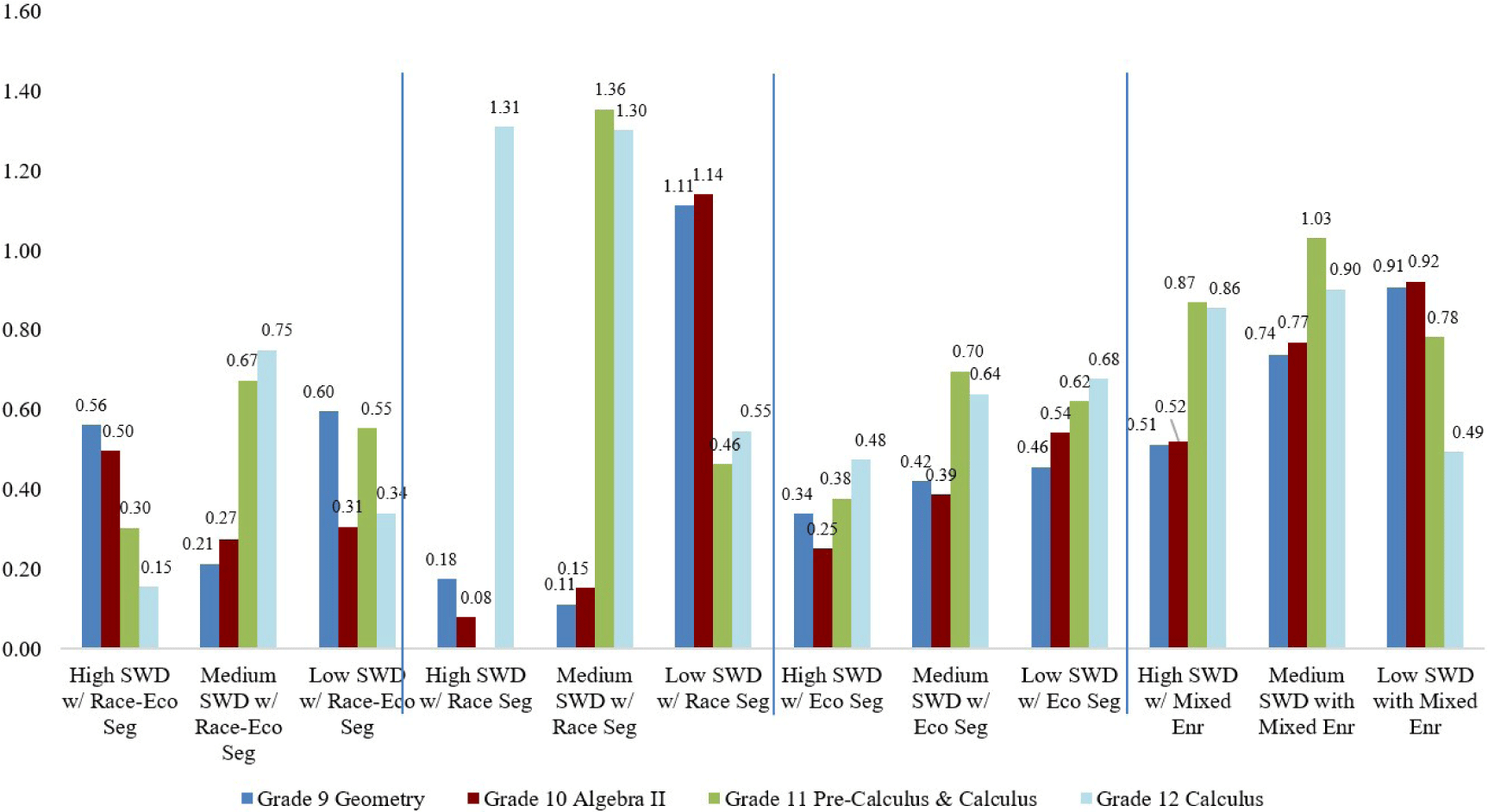
When comparing the intersection of low EL schools and White isolated schools with other EL/race/socioeconomic school composition types, we find that there is a disparity in advanced mathematics course enrollment in medium and high EL schools that are also segregated by race and/or income (Fig. 4). Consistent with our other findings, schools that are segregated by race continue to be an anomaly which appears to be linked to a small number of schools enrolling majority Asian students and having highly accelerated mathematics trends. In the case of high EL schools, it is possible that this group is also classified as ELs. However, there are fewer disparities for ELs in mixed enrollment schools suggesting that they could be benefiting from the general higher enrollment in advanced mathematics coursework in these schools.
In this section we review mathematics course-taking trends by school composition of SWDs. In 2019–2020, the number of students aged 3–21 who received special education services under the IDEA was 7.3 million, or 14% of all public-school students across the country (NCES, 2021). In New Jersey, 227,963 students or 17% received special education services in public schools in 2020 (NJDOE, 2020).
We found that for 9th grade, as SWD composition decreases, enrollment in an accelerated mathematics sequence becomes more likely - 31% of freshmen in low SWD schools are already taking Geometry compared to 26% in medium SWD and 18% in high SWD schools (Table 6). In schools with high percentages of SWDs, almost three quarters of students are enrolled in Algebra I (71%) but less than a quarter in Geometry (18%), suggesting a standard mathematics course-taking approach in these schools. The trends for medium and low SWD schools mirror the trends by schools segregated economically or both racially and economically.
In 10th grade, schools with high percentages of SWDs are almost as likely to enroll in Geometry (64%), the standard course for 10th grade, as are medium SWD schools (59%) (Table 6). The difference is in the percentage of students which have moved ahead to take Algebra II – 21% in high SWD schools compared to 29% in medium SWD schools and 32% in low SWD schools. With 87% of 10th graders in high SWD schools taking Geometry or Algebra II and 89% doing so in medium SWD schools, 10th grade mathematics course-taking is standard to advanced. The same is true for low SWD schools, though these schools lean toward a more advanced sequence with another 6% of 10th graders taking Pre-Calculus.
The gap widens in 11th grade when schools with low percentages of SWDs are more likely to enroll in higher-level mathematics courses than schools with medium and high percentages. For example, in schools with low percentages of SWDs almost half are enrolled in Algebra II (43%), over a quarter in Pre-Calculus (27%) and almost 10% are enrolled in Calculus (not shown in Table 6). However, in schools with medium and high SWD, that slight shift to Calculus in 11th grade has not occurred (5% and 2% respectively).
Finally, in 12th grade, schools with low and medium percentages of SWDs continue the pathway to Calculus and Statistics but schools with high percentages of SWDs are more likely to enroll in ‘Other Math’ courses. For example, 44% of seniors in schools with low percentages of SWDs are enrolled in Calculus or Statistics and 38% in schools with medium percentages of SWDs, but less than a quarter (23%) in high SWD schools.
In sum, the course-taking patterns suggest that as the percentage of SWDs increases in secondary schools, it is less likely that those enrolled will be on accelerated mathematics pathways. This pattern reflects the trend for schools segregated racially and economically so that by 11th and 12th grade, proportionally fewer students have shifted to advanced courses such as Pre-Calculus and Calculus. In contrast, students in low SWD schools appear to have a clear advantage. Again, unfortunately, we cannot know the courses that SWDs are taking from these data.
We see in the disparity ratios that schools with medium percentages of SWDs are almost as likely to enroll in Geometry in 9th grade as those in schools with low percentages of SWDs (Fig. 5). However, schools with high percentages of SWDs are far less likely to enroll in the course. The same is true for Algebra II in 10th grade though the likelihood is higher. In 11th grade, students in schools with medium percentages of SWDs are almost as likely to enroll in Pre-Calculus and Calculus as those with low percentages of SWDs. However, schools with high percentages of SWDs are only about half as likely to enroll in the course. The same is true in 12th grade with Calculus though the likelihood is smaller for those in schools with high percentages of SWDs.
When comparing the intersection of low SWD schools and White isolated schools with other SWD/race/socioeconomic school composition types, we find that low and medium SWD schools are more likely to enroll in high leverage mathematics courses than those with high proportions of SWDs (Fig. 6). Consistent with our findings for ELs, schools that are segregated by race continue to have distinctly different outcomes.
Despite the evident disparities in enrollment in high-leverage mathematics courses in NJ, there are several positive outliers - segregated schools which enroll students in advanced mathematics courses at rates much higher than the average for their category. A few positive outliers which are regular public schools with no special admission criteria or magnet program are highlighted here. These are schools which are managing to create advanced mathematics learning opportunities in segregated conditions where students typically do not have the opportunity to participate. Note that for the purposes of this study we focus on mathematics enrollment trends but the schools may not be positive outliers on other metrics. The four schools shown have enrolled more than 25% of their juniors in Pre-Calculus since 2016–2017, on par with non-segregated schools (see Table 7). Malcolm X Shabazz High School - a comprehensive school in the Newark Public Schools - and Hillside High School in Union County had 28% of their students enrolled in Pre-Calculus in their junior year in 2017. The other two secondary schools had slightly higher rates, enrolling more than 30% of their juniors in Pre-Calculus - Pennsauken High School (31%) and Dover High School (31%). These schools all serve students experiencing economic disadvantage of over 50% with two at almost 70%. They differ somewhat in the racial groups that they serve. For example, Dover High School serves 85% Latino students while Malcolm X Shabazz High School serves 89% Black students. The population of Pennsauken High School is more evenly distributed with 46% Latino, 32% Black, 10% White and 12% Asian students. With the exception of one school, they serve the state average of 7% EL students and Dover High School serves double that at 14% surpassing the national average. Two of the schools also serve more than the national average of SWDs suggesting that it is possible to enroll students in advanced mathematics courses in a variety of different contexts. In addition, three of the four schools had four year graduation rates of over 80% and one matched the state rate of 91% in 2020. There are also three schools that had postsecondary enrollment rates of over 50% in 2017 and two in 2020. It should be noted that postsecondary enrollment rates decreased during the COVID-19 pandemic (National Student Clearinghouse, 2022). More research is needed to explore the policies and practices of these schools related to course enrollment.
Discussion
Overall, the findings suggest that students in segregated schools in the state of New Jersey do not have the same levels of enrollment in advanced mathematics coursework as those in non-segregated secondary schools. In addition, students in schools with large concentrations of ELs and SWDs are taking high leverage mathematics coursework at lower rates. As such, students in segregated schools are not enrolled in the courses they need to be on track to graduate with an advanced diploma. As demonstrated in the Freshman on Track research, the freshman year is critical to student success (Allensworth, 2013). In our research, we found that students in mixed enrollment schools are already further along in the mathematics sequence than those in segregated schools in 9th grade. They are taking Geometry when students in segregated schools are largely still taking Algebra I. This continues throughout secondary school so that students in segregated schools fall further and further behind leading to only a small number being able to take Pre-Calculus and Calculus in their senior year. Further, students in segregated schools are more likely to enroll in “Other math” courses which do not necessarily count toward graduation. More research is needed to determine what comprises these “Other math” courses and how students make choices about course enrollment in the state.
As demonstrated here, the distribution of higher-level coursework is not equitable throughout the state such that schools that serve predominantly low-income students of color as well as ELs and SWDs do not have the same levels of enrollment as those that serve higher-income White students. In their framework, Kim et al. (2021) define equity as both the quantity or distribution of programs available to students with respect to their number, length, and intensity as well as the quality or the distribution of program type, efficacy, and rigor relative to the urbanicity and demographic composition of communities. We find that the distribution of mathematics learning opportunities in terms of intensity or enrollment rates and rigor or accelerated trajectories likely to lead to advanced course-taking is not equitable in segregated schools.
It is possible that schools with large Latino populations also may have high concentrations of ELs who are not given the opportunity to take high-leverage math courses (Thompson, 2017). In research about ELs in California (Hill, 2018; Reyes & Hwang, 2021), ELs who are not reclassified by high school, do not have room in their schedule to take other courses because they have to take required courses for EL programs. The same can be true of SWDs. For example, students identified with learning disabilities experience markedly lower levels of mathematics achievement than those who are not identified with a learning disability (Shifrer & Callahan, 2010). SWDs are highly underrepresented in STEM courses due to low expectations, limited exposure to prerequisite courses, lack of role models, and lack of access to individualized supports (Dunn et al., 2012). Since the concentration of SWDs in schools appears to be a disadvantage for enrollment in higher-level mathematics courses, efforts could be made for greater inclusion in those courses and in schools in general (Roos, 2019).
The fact that there are several positive outliers suggests that increasing participation in higher-level mathematics courses is a possibility for schools. These schools serve large populations of low-income students of color and have high rates of enrollment in advanced mathematics coursework. Students in these schools have the opportunity to graduate with a diploma that can put them on the path to be successful in higher education and the job market. The practices and policies in the positive outlier schools can be shared with other schools and districts as those seeking to improve carefully consider options to leverage. Some promising practices such as high dosage tutoring, peer tutoring, extended instructional time, and teacher professional development to support students once enrolled in higher-level mathematics coursework can also be considered. Importantly, the findings underscore the importance of work to desegregate and generally move away from systems that lead to isolation. We further suspect that low expectations plague the opportunities available to traditionally marginalized students in segregated or isolated contexts. Thus, coupled with efforts for inclusivity or integration, policies generating greater access to advanced opportunities, that are based on high expectations should be implemented in all schools.
This landscape study allows us to describe what mathematics course taking looks like across the state for different students. In this way, our research allows us to more deeply explain what segregation means for students’ lived experiences in schools. Similar to previous studies (Tractenberg et al., 2013), we explore course enrollment by levels of segregation. We explore disparities in course-taking and see that advanced mathematics course enrollment looks different in racially segregated schools vs. racially and economically segregated schools. We add to this literature by including schools with low, medium and high percentages of ELs and SWDs in order to see how mathematics course taking may differ for these groups. We find that high EL schools are almost just as likely as White isolated schools to enroll in Calculus in 12th grade. However, the same is not true for high SWD schools. It is important to remember that most ELs reclassify by secondary school and so high EL schools are a unique group that may be serving newcomer students with interrupted education (USDOE, 2017). In future research, we hope to explore what this might mean for postsecondary school enrollment and persistence.
Conclusion
New Jersey is a local control state in which it is left to the school districts to decide which mathematics curricula and course sequences to offer. However, it is also a highly segregated state in which schools that serve large numbers of low-income students of color, ELs and SWDs have far lower enrollment rates in advanced coursework. If that context is not recognized, local control can result in further restricting access to higher level coursework for these students. While we do not recommend that the state mandate the offering of higher-level mathematics coursework such as Algebra I in middle school, we do suggest that incentivizing districts to offer the course earlier could make a positive impact across the state particularly for students in highly segregated schools.
There is a growing trend in various parts of the country to begin offering Algebra in secondary school rather than middle school in an effort toward equity (Fortin, 2021). However, within a segregated educational context such as in New Jersey, we found that students in schools that serve low-income and low-income students of color, ELs and SWDs are restricted from being able to pursue more higher-level courses as they continue through secondary school. As found in our research, students in White isolated schools continue to have access to these courses earlier than those in segregated schools. It is also important to mention that even when they do not have access to these courses at school, they are able to find them elsewhere through private costly tutoring and extracurricular courses (Gamerman, 2023) furthering already existing equity gaps. Thus, it is critical that public schools offer advanced mathematics courses and prepare students to be successful in them.
Work such as this that is done in conjunction with the New Jersey State Policy Lab can translate into policy discussions that are grounded in research and data. These kinds of collaborations can have an impact across the state in showing a variety of stakeholders such as state legislators, policymakers, families, students and school and district personnel the disparities that still exist in mathematics course enrollment. We have been able to share this work with state leaders, legislators and in equity working groups. The New Jersey State Policy Lab provides a space to continue to share the landscape of mathematics education in the state. Part of the dialogue we hope to see in NJ going forward is that of increasing access and enrollment in advanced mathematics courses as well as better preparing and supporting students for success in those courses once enrolled.
